This page is linked from here; go there for some background.

These are photos of the first in a sequence of five 7-vertex deltahedra. I do not know what official name this one has, but I'll call it a "twisted augmented tetrahedron". Or just "7V1".

But there are actually TWO of these, one being a reflection of the other.

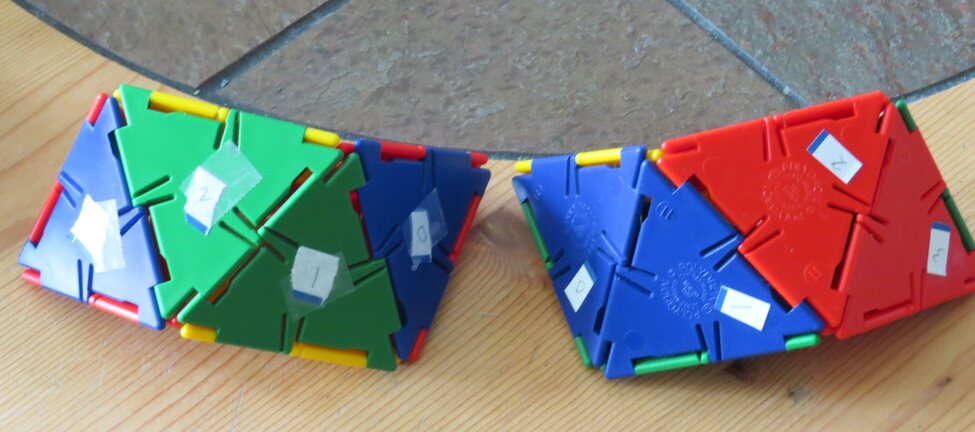
I apologize that the colorings in the left and right versions are different, but I couldn't decide (and still cannot) what is best when only four colors are available. More colors would have made things worse, anyway. I use the colors on the left for the animations and other images on the linked page.

But maybe the following colors are better! Here is why I call this thing a twisted augmented tetrahedron...

Take a pair of tetrahedra and stick them together; do that twice:

There are essentially two ways to stick the pairs together. Here is the first, which gives the twisted augmented tetrahedron. Get ready to snap the two pairs together by lining them up like so:

Okay, in my case (since I'm using Polydron pieces), get rid of the two faces that will get snapped:

And close it up.

Earlier, I said "essentially". That's because you can make an alternate, reflected version by twisting the other way, before grafting the pairs. This makes this twisted critter the most complicated of the five in some respects.

The second "essential" way is to use the third possibility of attaching the pairs — there are really three "twists" possible — the two above and the one below, which gives us the second in the sequence of 7-vertex deltahedra:

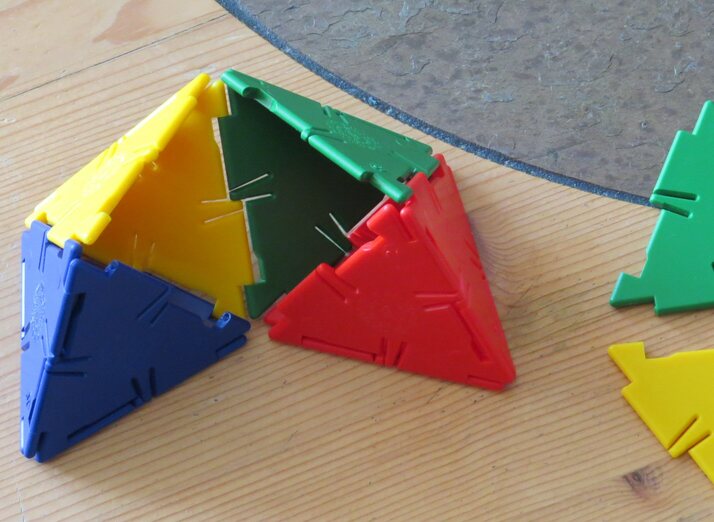
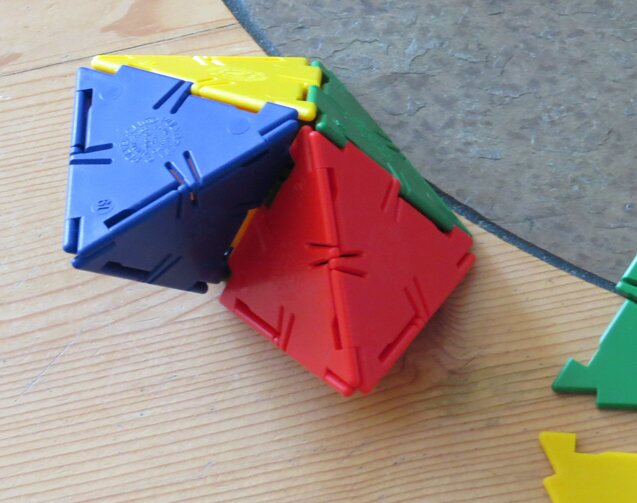
The one above ("7V2") actually has the official name "augmented tetrahedron", which is why I call 7V1 a "twisted" version of that. See the various versions of the "augmented tetrahedra" on Wikipedia (ours is the one with 10 triangles). The name is a bit strange to me, since I see it as pasting together four tets, rather than augmenting just one. If you look at that Wikipedia page (and mine, HERE), you'll see the "augmented octahedron", the fifth in the series. Okay, it is made by "augmenting" an octahedron by adding a tetrahedron. But then it can also be seen as augmenting a tetrahedron by adding an octahedron!

That reminds me of the joke about the guy who wakes up with a frog attached to his head. He goes to the doctor. The doc asks, "What seems to be the problem?" The frog says, "Doc, I woke this morning with this abscess on my butt." So is that an augmented human or an augmented frog? (It's twisted, either way.)

In any case, an infinite number of deltahedra can be made by snapping together tetrahedra. In the set of five we are looking at here, it turns out that this yields just three: the twisted one featured here (7V1), the augmented tet (7V2) above, and what I call the "triple augmented tetrahedron" (so named for reasons as above) or "7V3", which had 3-fold rotational symmetry and is here.

There are five ways (I think!) to add a tetrahedron to the twisted augmented tetrahedron (7V1) to get a new (8-vertex) deltahedron. Which ones among the following 14 are they? (Source for image: this paper by N. Tsuruta, J. Mitani, Y. Kanamori, Y. Fukui (2015), Random Realization of Polyhedral Graphs as Deltahedra, Journal for Geometry and Graphics, Volume 19 (2015), No. 2, 227–236.)