This page is linked from here; go there for some background.

This is the "pentagonal bipyramid" (I'll also call it "7V4"),

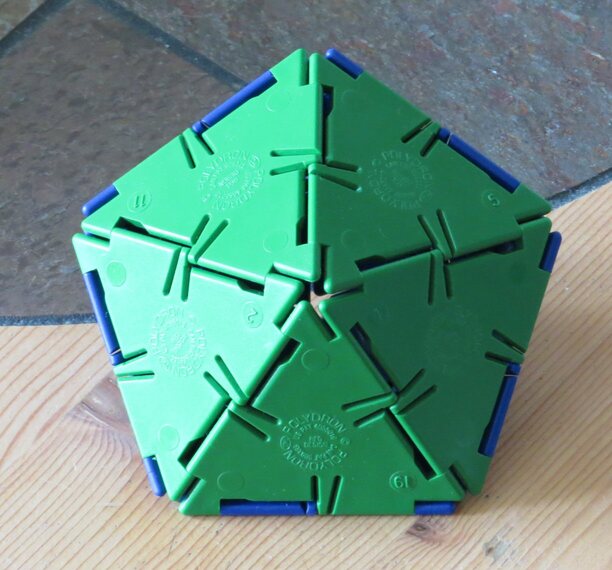
which is what you get if you snap together two pentagonal pyramids.

If you consider how the augmented tetrahedron 7V2 can be built by gluing together tetrahedra, then you might wonder if you can just add one last tetrahedron to create this bipyramid.

NOPE. Here is what you get when you do add a regular tet to that tempting gap — it's another "augmented tetrahedron", but with 8 vertices (12 triangles); it is one the family of the 14 distinct 8-vertex deltahedra, number 6 in the list below.

(The previous two images are ripped from the Wikipedia page for deltahedra.)

Thus the family of deltahedra cannot be built from only regular tetrahedra — the pentagonal bipyramid is a different fundamental form. So is the augmented tetrahedron 7V5.

In view of the failure of the above equality, one can ask, Is there a chain of tetrahedra that makes a closed loop? The answer here is also negative. But you can get as close as you like. See the 2016 paper by Michael Elgersma and Stan Wagon: The Quadrahelix: A Nearly Perfect Loop of Tetrahedra

There is just one way to add a tetrahedron to the pentagonal bipyramid (7V4); you get the 9th in the following list of the 14. (Source: this paper by N. Tsuruta, J. Mitani, Y. Kanamori, Y. Fukui (2015), Random Realization of Polyhedral Graphs as Deltahedra, Journal for Geometry and Graphics, Volume 19 (2015), No. 2, 227–236.

Which ones are built of tets alone? Which are from tets and either the bipyramid or the augmented tetrahedron 7V5? What other fundamental figures must be used? (Number 7, for sure...)